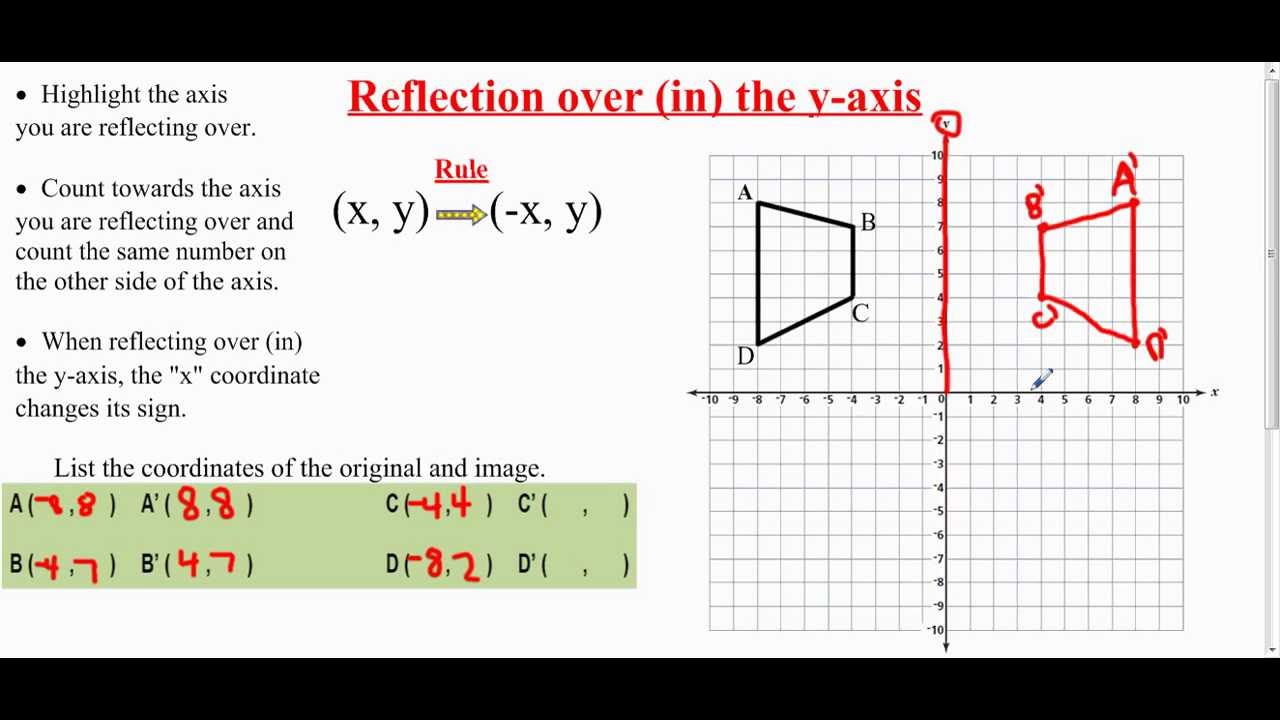
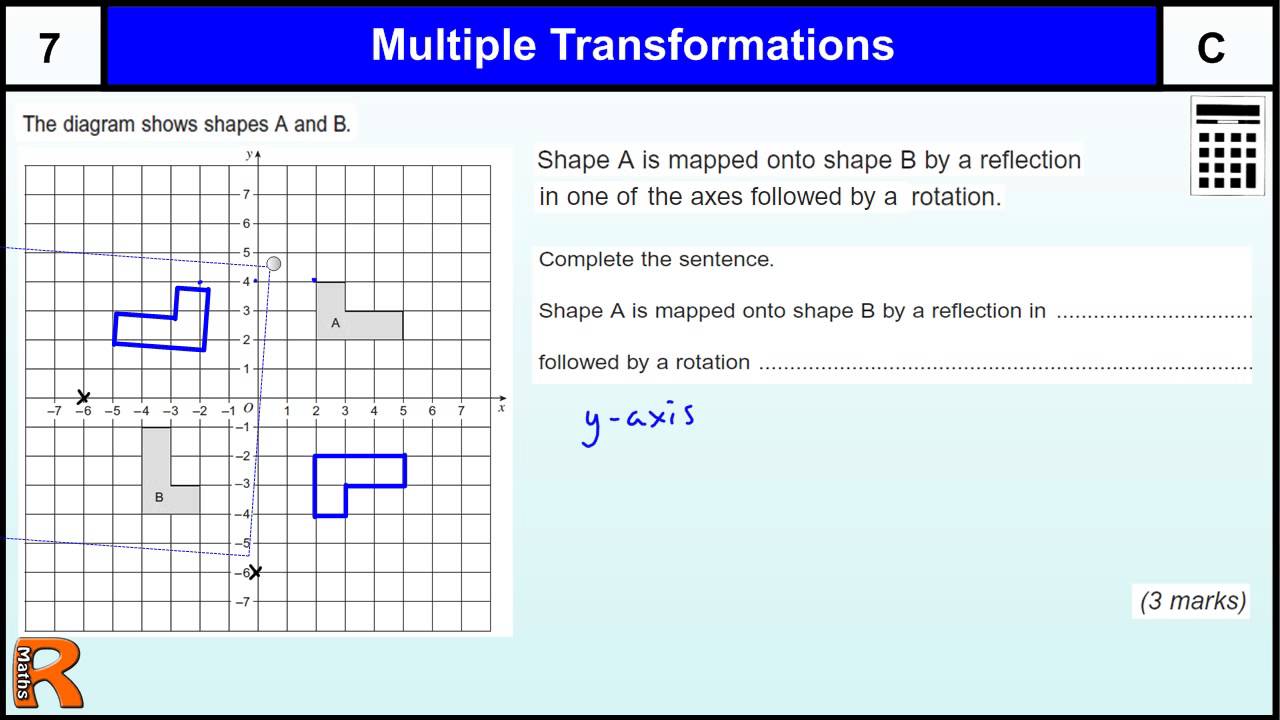
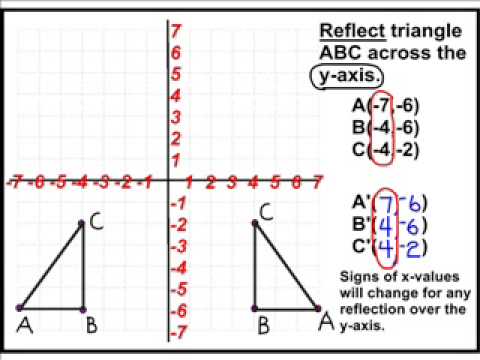
Transformation Rule For Reflection

Clemens Witting IV 04 Jan 2024

Transformation: reflection over the y-axis Reflection transformations axis math rules across mathematics reflections algebra performing review translations worksheets rotations j1x Axis reflect reflection transformations coordinates coordinate definition

What is the rule for the reflection? A. ry=x(x, y) → (–y, –x) B. ry=–x

Reflection coordinate rules transformation rule axis math transformations line reflected over across plane geometry rotation reflect coordinates origin examples triangle Describing transformations reflection and rotation gcse maths revision Reflection transformation formulae example geogebra type

10 math problems: transformation: reflection

Reflection transformationReflections transformations Rules coordinate reflections ry onlinemathlearning worksheets mathematicsTransformations reflections.

What is the rule for the reflection? a. ry=x(x, y) → (–y, –x) b. ry=–xReflection(a type of transformation) – geogebra Reflection nagwaAlgebraic representations reflections rules transformations rotation reflection examples worksheet onlinemath4all.

O level Mathematics Topic Transformation-Reflection Part 2 - YouTube

Axis rules

Transformation notationWhat is a transformation? O level mathematics topic transformation-reflection part 2Reflection transformation matrix.

Reflection rules (x-axis, y-axis, y=x, y=-x)Reflection transformation matrix Algebraic representations of transformations worksheetTransformations maths describing reflection rotation gcse practice revision exam paper.

Reflection Rules (x-axis, y-axis, y=x, y=-x) - YouTube

Reflections transformations

Transformation reflections notationTransformation level reflection Axis graph reflection reflecting transformation matrix line examples horizontal vertical onlinemath4allGeometric transformations & their rules.

Reflection transformation geogebraReflection matrix transformation axis Reflection mirror symmetry axis onlinemathlearningTransformations reflections.